Energy of Phase Changes
Below is a pure molecular substance at a low temperature in the solid phase. As the temperature increases the substance will change phase to liquid and then to gas. The temperature where the phase chane occurs depends on the strength of the intermolecular attractive forces (labled here as Van der Waals attractions). Since the particles appear to be nonpolar we assume the only intermolecular attractive force is London Dispersion forces.
For a particulate level view of phase changes we will look at a model developed by concord.org. In this model heat is added to the sample when the temperature is increased, or removed from the system when the tmperature is lowered. While a sample is in the same phase (gas, liquid or solid) the amount of heat added can be calculated by using the relationship q (heat) = mass*c*∆T. During a phase change, since the ∆T = 0 the amount of heat is determined by the relationship q(heat) = ∆H(phase change)°amount of substance. The total heat required depends on the the number of phase changes that the sample experiences plus the the number of phases that are present during the overall temperature changes. |
|
Below is a plot of what happens to the temperature of a sample of water as
heat was added at a constant rate. The heating curve is summarized in the graph
below;
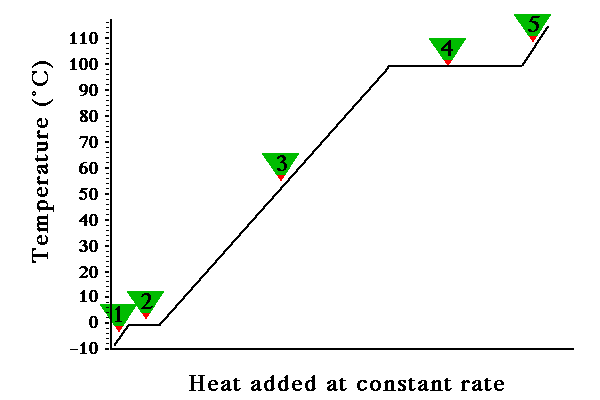
We notice the obvious, as heat is added (along the x-axis) the temperature
of the sample, in general, increases. Looking at the plot more closely we actually
see there are two plateaus where the temperature remains constant as heat is
added. The sample is water so it is interesting that the two plateaus are at
0 degrees Celsius and 100 degrees Celsius. One plateau (#2) corresponds to the
melting/freezing point of water, the other plateau (#4) corresponds to the boiling/condensing
point of water. Besides the two plateaus there are three regions where the temperature
increases linearly with added heat.
The five label regions can be characterized by specifying the temperature
change (if any) and the phase(s) present.
At #1 the temperature changes from -10 degrees Celsius to 0 degrees Celsius;
the phase is H2O(s). We are referring to the line with a positive
slope between - 10 deg Celsius and 0 deg Celsius. So as heat is added the temperature
of the sample of water increases linearly. That the line has a constant slope
over the temperature range suggests uniform behavior. That is there is a relationship
between the amount of heat added and the temperature change. It turns out that
how much heat is required to change the temperature of solid water also depends
on the amount of water. The characteristic property that relates the amount
of heat required to change the temperature of 1 gram of a substance by 1 degree
Celsius is called specific heat. The specific heat of water in the solid phase
has a value of 2.09 J g-1 C-1.
At #2 the temperature does not change, but heat is added. The added heat is
absorbed by the solid converting the solid to the liquid. So at the left most
point on the horizontal line the sample is all solid and the temperature is
0 degrees Celsius. As heat is added the solid melts, recall it takes energy
to over come the attractive forces holding the particles together in the solid
phase, forming the liquid. The amount of intermolecular attractive forces broken
in this phase change is small since the particles are very close to each other
in each phase. So at the right most point of the horizontal line the sample
is all liquid at 0 degrees Celsius. The heat required to convert water from
the solid phase to the liquid phase;
H2O(s) ---> H2O(l)
is called the heat (enthalpy) of fusion (heat of solidification). For water
the heat of fusion has a value of 6.01 kJ mol-1.
At #3 the temperature changes from 0 degrees Celsius to 100 degrees Celsius.
The phase is liquid. Again notice the linear behavior. In this temperature range,
given the amount of water remains constant, the addition of a given amount of
heat causes the temperature of the water to change by the same amount. The specific
heat of liquid water is 4.184 J g-1 C-1. Comparing liquid
water to solid water it takes more than twice the amount of heat to change the
temperature of liquid water by the same amount compared to solid water.
At #4 the temperatrue does not change as the heat is added. At this plateau
the heat added is absorbed by the liquid as it is converted to the gas(vapor)
phase. The heat absorbed is used to overcome the attractive forces between the
particles in the liquid phase. Notice the amount of energy required to convert
a liquid to its gas is much greater compared to the energy required to convert
a solid to its liquid. At the left-most point on this line the sample is all
liquid at 100 degrees Celsius. Moving to the right the amount of water in the
liquid and vapor change, and the temperature remains constant. At the extreme
right the sample is all vapor at 100 degrees Celsius.The heat required to convert
water from the liquid phase to the gas phase;
H2O(l) ---> H2O(g)
is called the heat (enthalpy) of vaporization (heat of condensation). For
water the heat of vaporization has a value of 40.67 kJ mol-1.
At #5 the temperature changes from 100 degrees Celsius to 110 degrees celsius.
The phase is gas(vapor). In the vapor phase the specific heat of water is 1.84
J g-1 C-1.
Here is a summary table of the specific heats and enthalpy of phase changes
for water;
Specific Heat of H2O(s) |
2.09 J g-1 C-1 |
Specific Heat of H2O(l) |
4.184 J g-1 C-1 |
Specific Heat of H2O(g) |
1.84 J g-1 C-1 |
Enthalpy of fusion |
6.01 kJ mol-1 |
Enthalpy of vaporization |
40.67 kJ mol-1 |
Let's look at several sample problems;
How much heat is required to change 36.0 g of H2O(l) at 100 deg
C to 36.0 g of H2O(g) at 100 deg C? Answer
How much heat is required to convert 30.0 g of H2O(s) at -10 deg
C to 30.0 g of H2O(g) at 110 degC. Answer