Chapter 13
Colligative Properties
Having discussed concentration units we need to extend the idea of solutions
to investigate how some physical properties of the solvent are effected upon
addition of a nonvolatile solute. A nonvolatile solute is a solute which has
little tendency to escape from the solution. A solution of a nonvolatile solute
(solid) has properties that are modified from the properties of the liquid (solvent).
The difference between the properties of the solution and those of the pure
liquid are a consequence of the number of solute particles present in the solution.
The properties which depend on the number of particles of solute are called
colligative properties.
These include;
1) Vapor Pressure lowering
2) Boiling Point elevation
3) Freezing Point depression
4) Osmotic Pressure
Colligative properties are properties which depend on the number of molecules
or ions of solute present, and not on what the particles are (as long as they
are not volatile). We will find colligative properties are important as they
will provide us with information about the number of particles of solute present,
and hence about molecular weights and degree's of dissociation (ionization)
in solution. Early chemists, such as Arrhenius, were able to show that for some
solutes more particles were present in solution than there were 'molecules'
of solute, and hence that the solute particles were breaking (dissociating)
into ions.
To understand the effect of a solute on the physical properties of a liquid
we will begin with what happens to the vapor pressure
(Pre-Lecture Exploration
#9 Expert's Response)of a pure liquid upon addition of a solute. A pure
liquid, at a given temperature will be in equilibrium with its vapor. If we
consider water at 25 ŻC, the vapor pressure is 23.6 Torr. If we prepare solutions,
by adding a nonvolatile solute, containing different mole fractions of solvent
as shown below, and measure the vapor pressure due to water above the solution,
we would obtain the following data.
Mole Fraction (solvent)
|
Vapor Pressure (mm Hg)
|
1.0
|
23.600
|
.9
|
21.240
|
.8
|
18.880
|
.7
|
16.520
|
.6
|
14.160
|
.5
|
11.800
|
.4
|
9.440
|
.3
|
7.080
|
.2
|
4.720
|
.1
|
2.360
|
0
|
0
|
Graphing this data the plot looks like;
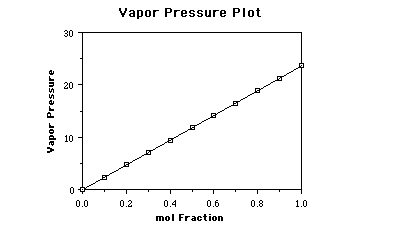
We discuss the Table and the graph in the Pre-Lecture
Exploration #10 Expert's Response. Review the exploration to see some discussion
of the relationship between vapor pressure and mol fraction of solvent.
This is called Raoult's Law and is given as;

where;
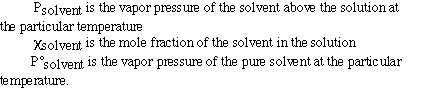
Here is a sample problem (here is the problem
from class on Wednesday, September 19, 2001) using Raoult's law;

The equation;

can be rewritten in a slighlty different form to suggest another
useful technique. The mole fraction of solvent is given as;
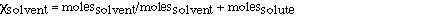
Which can be further transformed to;

From this relationship it is possible to determine the molar
mass of a compound from the change in vapor pressure of the solvent. (Here is
the problem from class on
Wednesday, September 19, 2001)

The total vapor pressure above a solution is the sum of the
vapor pressures of the solution components (solute and solvent).

Substituting for Raoult's law we have;
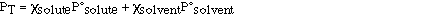
The composition of the vapor is not the same as the composition
of the solution. Remember vapor composition, while it does depend on the mole
fraction of the component of the solution also depends on the vapor pressure
of the components. The vapor above a solution is always richer in the component
that has the higher vapor pressure at the given temperature.
How the nonvolatile solute effects the vapor pressure of the
pure liquid can now be transferred to the phase
diagram to help us understand how the boiling point and melting point of
the solvent are changed. If we select a point on the liquid–vapor equilibrium
line of the pure solvent we can read a particular vapor pressure. If a nonvolatile
solute is added we know the vapor pressure is lowered by an amount that depends
on the concentration of the solute particles present. So for the solution the
liquid–vapor equilibrium line is below that of the pure liquid. If we continue
along the liquid–vapor line for the pure liquid we continue to plot a new line
for the solution. As we approach the boiling point, which occurs when the vapor
pressure is 760 mm and we added some nonvolatile solute the vapor pressure drops.
The solution stops boiling. To get the solution to boil we must increase the
temperature of the solution––the boiling point of the solution is higher than
that of the pure liquid. The boiling point is elevated by the addition of a
nonvolatile solute.
When we consider the effect on the melting point of the liquid
we must recall that melting occurs at a temperature when the pure liquid is
in equilibrium with the pure solid. In a solution, the liquid contains some
solute, which does not crystallize in the pure solvent solid structure. So not
every molecule that collides with the pure solid solvent is a solvent particle,
however, every molecule that melts is pure solvent. So more molecules are melting,
going into the liquid phase, than are solidifing. Inorder to re–establish the
equilibrium the temperature of the solution must be lowered. At the lower temperature
the solvent particles are moving slower and are more likely to solidify. The
result is that the melting point of the liquid is depressed by the addition
of a nonvolatile solute.
Now let's consider the boiling point and the freezing point of
the solution. Let's look at the phase diagram for water. We know the location
of the normal boiling point and the normal freezing point for water on the phase
diagram. If we plot the liquid vapor equilibrium line for a solution of constant
concentration (mol fraction) the new vapor pressure curve of the solution looks
like this.
The resulting solution has a new freezing point which lower than
the original freezing point. When the liquid freezes only the solvent molecules
are formed in the solid, so no new solid-vapor equilibrium line is formed. The
freezing point of the solution is depressed below the normal freezing point.
If we turn our attention to the boiling point of the solution
we see the normal boiling point is elevated as a result of the solute. We can
understand the boiling point elevation in the following terms. At the normal
boling point of the pure solvent the vapor pressure is equal to the atmospheric
pressure. If a solute is added the vapor pressure is lowered. In order for the
solution to boil the temperature must be increased to increase the vapor pressure
of the solution until it again equals atmospheric pressure.
It turns out that for dilute solutions of nonvolatile solutes
the  Tfp and the
Tfp and the  Tbp
are proportional to the molality of the solution.
Tbp
are proportional to the molality of the solution.
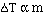
to get an equality we add a constant and
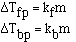
The magnitude of the constants are different for a particular
solvent and also vary with different solvents. The units on 'k', the freezing
point constant or the boiling point constant, are degrees C m-1.
For water the freezing point constant is 1.86 degrees C m-1 and the
boiling point constant is 0.512 degrees C m-1. A 1 molal aqueous
solution of any nonvolatile nonelectrolyte boils at 100.512 degrees C and melts
at –1.86 degrees C. So if we have a concentration of a nonvolatile solution
other than 1 molal we can use either equation to calculate the new boiling or
freezing point. It must be remembered that when solving for ∆T that if the freezing
point is expected the ∆T must be subtracted from 0 degrees C (freezing point
depression) and added to 100 degrees C (boiling point elevation).